G2lib Namespace Reference

Clothoids: G2lib Namespace Reference

Clothoids

Classes
class	AsyPlot

class	BaseCurve

class	BBox

class	Biarc

class	BiarcList

class	CircleArc

class	ClothoidCurve

class	ClothoidList

class	ClothoidSplineG2

class	Dubins

class	Dubins3p

class	G2solve2arc

class	G2solve3arc

class	G2solveCLC

class	LineSegment

class	PolyLine
	Class to manage a collection of straight segment. More...

class	Solve2x2

class	Triangle2D

Typedefs
using	istream_type = std::basic_istream<char>
	input streaming

using	ostream_type = std::basic_ostream<char>
	output streaming

using	real_type = double
	real type number

using	integer = int
	integer type number

using	AABB_TREE = Utils::AABBtree<real_type>
	`AABB` tree type

using	AABB_SET = Utils::AABBtree<real_type>::AABB_SET
	Set type used in `AABB` tree object.

using	AABB_MAP = Utils::AABBtree<real_type>::AABB_MAP
	Map type used in `AABB` tree object.

using	GenericContainer = GC_namespace::GenericContainer
	Generic container object.

using	CurveType

using	Ipair = std::pair<real_type,real_type>
	Pair of two real number.

using	IntersectList = std::vector<Ipair>
	Vector of pair of two real number.

using	DubinsType

using	Dubins3pBuildType

Functions
bool	build_guess_theta (integer n, real_type const x[], real_type const y[], real_type theta[])

ostream_type &	operator<< (ostream_type &stream, Biarc const &bi)

ostream_type &	operator<< (ostream_type &stream, BiarcList const &CL)

ostream_type &	operator<< (ostream_type &stream, CircleArc const &c)

ostream_type &	operator<< (ostream_type &stream, ClothoidCurve const &c)

ostream_type &	operator<< (ostream_type &stream, ClothoidSplineG2 const &c)

ostream_type &	operator<< (ostream_type &stream, ClothoidList const &CL)

string	to_string (CurveType n)

CurveType	curve_promote (CurveType A, CurveType B)

bool	collision (BaseCurve const pC1, BaseCurve const pC2)

bool	collision_ISO (BaseCurve const pC1, real_type offs_C1, BaseCurve const pC2, real_type offs_C2)

bool	collision_SAE (BaseCurve const pC1, real_type offs_C1, BaseCurve const pC2, real_type offs_C2)

void	intersect (BaseCurve const pC1, BaseCurve const pC2, IntersectList &ilist)

void	intersect_ISO (BaseCurve const pC1, real_type offs_C1, BaseCurve const pC2, real_type offs_C2, IntersectList &ilist)

void	intersect_SAE (BaseCurve const pC1, real_type offs_C1, BaseCurve const pC2, real_type offs_C2, IntersectList &ilist)

ostream_type &	operator<< (ostream_type &stream, BBox const &bb)

integer	to_integer (DubinsType d)

bool	Dubins_build (real_type x0, real_type y0, real_type theta0, real_type x1, real_type y1, real_type theta1, real_type k_max, DubinsType &type, real_type &L1, real_type &L2, real_type &L3, real_type grad[2])

string	to_string (DubinsType n)

Dubins3pBuildType	string_to_Dubins3pBuildType (string const &str)

void	FresnelCS (real_type x, real_type &C, real_type &S)

void	FresnelCS (integer nk, real_type x, real_type C[], real_type S[])

void	GeneralizedFresnelCS (integer nk, real_type a, real_type b, real_type c, real_type intC[], real_type intS[])

void	GeneralizedFresnelCS (real_type a, real_type b, real_type c, real_type &intC, real_type &intS)

real_type	Sinc (real_type x)
	\( \frac{\sin x}{x} \)

real_type	Sinc_D (real_type x)
	\( \frac{\mathrm{d}}{\mathrm{d}x} \frac{\sin x}{x} \)

real_type	Sinc_DD (real_type x)
	\( \left(\frac{\mathrm{d}}{\mathrm{d}x}\right)^2 \frac{\sin x}{x} \)

real_type	Sinc_DDD (real_type x)
	\( \left(\frac{\mathrm{d}}{\mathrm{d}x}\right)^3 \frac{\sin x}{x} \)

real_type	Cosc (real_type x)
	\( \frac{1-\cos x}{x} \)

real_type	Cosc_D (real_type x)
	\( \frac{\mathrm{d}}{\mathrm{d}x} \frac{1-\cos x}{x} \)

real_type	Cosc_DD (real_type x)
	\( \left(\frac{\mathrm{d}}{\mathrm{d}x}\right)^2 \frac{1-\cos x}{x} \)

real_type	Cosc_DDD (real_type x)
	\( \left(\frac{\mathrm{d}}{\mathrm{d}x}\right)^3 \frac{1-\cos x}{x} \)

real_type	Atanc (real_type x)
	\( \frac{\arctan x}{x} \)

real_type	Atanc_D (real_type x)
	\( \frac{\mathrm{d}}{\mathrm{d}x} \frac{\arctan x}{x} \)

real_type	Atanc_DD (real_type x)
	\( \left(\frac{\mathrm{d}}{\mathrm{d}x}\right)^2 \frac{\arctan x}{x} \)

real_type	Atanc_DDD (real_type x)
	\( \left(\frac{\mathrm{d}}{\mathrm{d}x}\right)^3 \frac{\arctan x}{x} \)

void	rangeSymm (real_type &ang)

void	minmax3 (real_type a, real_type b, real_type c, real_type &vmin, real_type &vmax)

real_type	projectPointOnCircleArc (real_type x0, real_type y0, real_type c0, real_type s0, real_type k, real_type L, real_type qx, real_type qy)

real_type	projectPointOnCircle (real_type x0, real_type y0, real_type theta0, real_type k, real_type qx, real_type qy)

bool	pointInsideCircle (real_type x0, real_type y0, real_type c0, real_type s0, real_type k, real_type qx, real_type qy)

integer	solveLinearQuadratic (real_type A, real_type B, real_type C, real_type a, real_type b, real_type c, real_type x[], real_type y[])

integer	solveLinearQuadratic2 (real_type A, real_type B, real_type C, real_type x[], real_type y[])

integer	intersectCircleCircle (real_type x1, real_type y1, real_type theta1, real_type kappa1, real_type x2, real_type y2, real_type theta2, real_type kappa2, real_type s1[], real_type s2[])

integer	is_counter_clockwise (real_type const P1[], real_type const P2[], real_type const P3[])

integer	is_point_in_triangle (real_type const point[], real_type const P1[], real_type const P2[], real_type const P3[])

void	xy_to_guess_angle (integer npts, real_type const x[], real_type const y[], real_type theta[], real_type theta_min[], real_type theta_max[], real_type omega[], real_type len[])

ostream_type &	operator<< (ostream_type &stream, Dubins const &bi)

ostream_type &	operator<< (ostream_type &stream, Dubins3p const &bi)

ostream_type &	operator<< (ostream_type &stream, LineSegment const &c)

ostream_type &	operator<< (ostream_type &stream, PolyLine const &P)

ostream_type &	operator<< (ostream_type &stream, Triangle2D const &t)

Detailed Description

file: BBox.cc

file: Biarc.cc

file: BaseCurve.hh

file: BBox.hxx

file: Biarc.hxx

file: BiarcList.hh

file: Circle.hxx

file: Clothoid.hxx

file: ClothoidList.hxx

file: Dubins.hxx

file: Dubins3p.hxx

file: Fresnel.hxx

file: G2lib.hh Clothoid computations routine namespace

'

file: Line.hxx

file: PolyLine.hxx

file: Triangle2D.hxx

file: Dubins.cc

file: Dubins3p.cc

file: Dubins3p_ellipse.cc

file: Dubins3p_pattern.cc

Typedef Documentation

◆ CurveType

using G2lib::CurveType

Initial value:

 enum class CurveType : integer {
    LINE,
    POLYLINE,
    CIRCLE,
    BIARC,
    BIARC_LIST,
    CLOTHOID,
    CLOTHOID_LIST,
    DUBINS,
    DUBINS3P
  }

Enumeration type for curve type

◆ Dubins3pBuildType

using G2lib::Dubins3pBuildType

Initial value:

 enum class Dubins3pBuildType : integer {
    SAMPLE_ONE_DEGREE,
    PATTERN_SEARCH,
    PATTERN_TRICHOTOMY,
    PATTERN_SEARCH_WITH_ALGO748,
    PATTERN_TRICHOTOMY_WITH_ALGO748,
    ELLIPSE,
    POLYNOMIAL_SYSTEM
  }

Type of Dubins for three points construction algorithm

SAMPLE_ONE_DEGREE search solution by sampling a fixed angles
PATTERN_SEARCH search solution by using pattern search
PATTERN_TRICHOTOMY search solution by using pattern search based on tricotomy
PATTERN_SEARCH_WITH_ALGO748 search solution by using pattern search and refinement using ALGO748
PATTERN_TRICHOTOMY_WITH_ALGO748 search solution by using pattern search with tricotomy and refinement using ALGO748
ELLIPSE search solution by using ellipse geometric construction
POLYNOMIAL_SYSTEM search solution by solving polinomial system

◆ DubinsType

using G2lib::DubinsType

Initial value:

enum class DubinsType : integer

{ LSL, RSR, LSR, RSL, LRL, RLR, DUBINS_ERROR }

G2lib::DubinsType

enum class DubinsType :integer { LSL, RSR, LSR, RSL, LRL, RLR, DUBINS_ERROR } DubinsType

Definition Dubins.hxx:44

Type of Dubins solution

LSL left-stright-left solution
RSR right-stright-right solution
LSR left-stright-right solution
RSL right-stright-left solution
LRL left-right-left solution
RLR right-left-right solution

Function Documentation

◆ build_guess_theta()

bool G2lib::build_guess_theta	(	integer	n,
		real_type const	x[],
		real_type const	y[],
		real_type	theta[] )

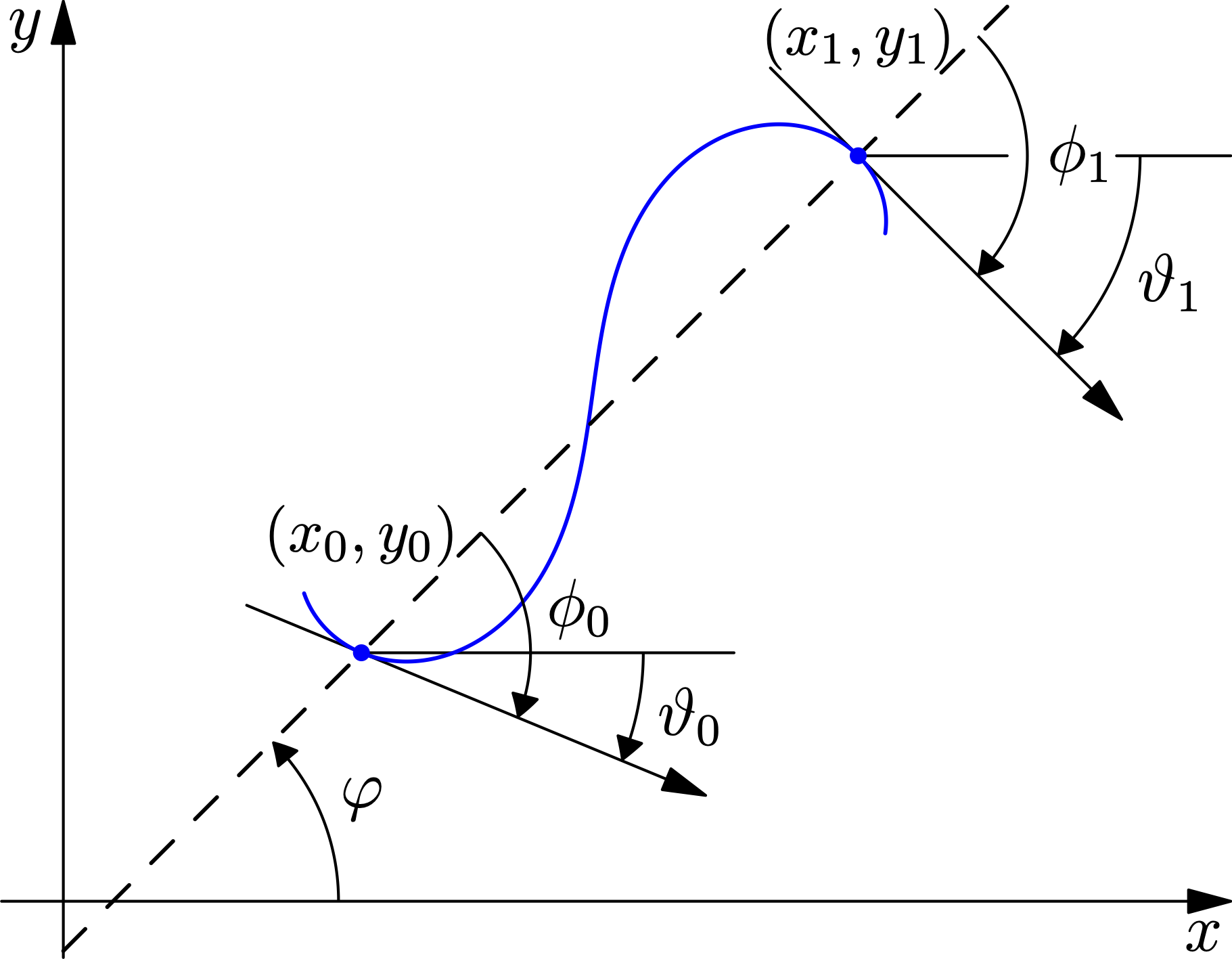
Given a list of points \( (x_i,y_i) \) build a guess of angles for a spline of biarc.

Parameters

[in]	n	number of points
[in]	x	\(x\)-coordinates
[in]	y	\(y\)-coordinates
[out]	theta	guessed angles

◆ collision()

bool G2lib::collision	(	BaseCurve const *	pC1,
		BaseCurve const *	pC2 )

Check curve collision

Parameters

[in]	pC1	first curve
[in]	pC2	second curve

Returns: true if the curves collide

◆ collision_ISO()

bool G2lib::collision_ISO	(	BaseCurve const *	pC1,
		real_type	offs_C1,
		BaseCurve const *	pC2,
		real_type	offs_C2 )

Check curve collision

Parameters

[in]	pC1	first curve
[in]	offs_C1	offset of the first curve
[in]	pC2	second curve
[in]	offs_C2	offset of the second curve

Returns: true if the curves collide

◆ collision_SAE()

bool G2lib::collision_SAE	(	BaseCurve const *	pC1,
		real_type	offs_C1,
		BaseCurve const *	pC2,
		real_type	offs_C2 )

inline

Check curve collision

Parameters

[in]	pC1	first curve
[in]	offs_C1	offset of the first curve
[in]	pC2	second curve
[in]	offs_C2	offset of the second curve

Returns: true if the curves collide

◆ curve_promote()

CurveType G2lib::curve_promote	(	CurveType	A,
		CurveType	B )

extern

Given two curve type determine curve type that cointain both type

Parameters

[in]	A	first curve type
[in]	B	second curve type

Returns: the curve type super type of both

◆ Dubins_build()

bool G2lib::Dubins_build	(	real_type	x0,
		real_type	y0,
		real_type	theta0,
		real_type	x3,
		real_type	y3,
		real_type	theta3,
		real_type	k_max,
		DubinsType &	type,
		real_type &	L1,
		real_type &	L2,
		real_type &	L3,
		real_type	grad[2] )

Given two points

\[ (x_0,y_0),\qquad (x_3,y_3) \]

two angles

\[ \theta_0,\qquad \theta_1 \]

and a maximum of curvature \( \kappa_{\max} \)

compute the solution of the Dubins problem

Parameters

[in]	x0	coordinate \( x_0 \)
[in]	y0	coordinate \( y_0 \)
[in]	theta0	angle \( \theta_0 \)
[in]	x3	coordinate \( x_3 \)
[in]	y3	coordinate \( y_3 \)
[in]	theta3	angle \( \theta_3 \)
[in]	k_max	max curvature \( \kappa_{\max} \)
[out]	type	solution type
[out]	L1	length of first arc
[out]	L2	length of second arc
[out]	L3	length of third arc
[out]	grad	gradient of the solution respect to initial and final angle

Returns: true if a solution is found

◆ FresnelCS() [1/2]

void G2lib::FresnelCS	(	integer	nk,
		real_type	x,
		real_type	C[],
		real_type	S[] )

Compute Fresnel integrals and its derivatives

\[ C(x) = \int_0^x \cos\left(\frac{\pi}{2}t^2\right) \mathrm{d}t, \qquad S(x) = \int_0^x \sin\left(\frac{\pi}{2}t^2\right) \mathrm{d}t \]

Parameters

nk	maximum order of the derivative
x	the input abscissa
S	S[0]= \( S(x) \), S[1]= \( S'(x) \), S[2]= \( S''(x) \)
C	C[0]= \( C(x) \), C[1]= \( C'(x) \), C[2]= \( C''(x) \)

◆ FresnelCS() [2/2]

void G2lib::FresnelCS	(	real_type	y,
		real_type &	C,
		real_type &	S )

Purpose:

Compute Fresnel integrals C(x) and S(x)

\[ S(x) = \int_0^x \sin t^2 \,\mathrm{d} t, \qquad C(x) = \int_0^x \cos t^2 \,\mathrm{d} t \]

Example:

\( x \)	\( C(x) \)	\( S(x) \)
0.0	0.00000000	0.00000000
0.5	0.49234423	0.06473243
1.0	0.77989340	0.43825915
1.5	0.44526118	0.69750496
2.0	0.48825341	0.34341568
2.5	0.45741301	0.61918176

Adapted from:

William J. Thompson, Atlas for computing mathematical functions : an illustrated guide for practitioners, with programs in C and Mathematica, Wiley, 1997.

Author:

Venkata Sivakanth Telasula, email: sivak.nosp@m.anth.nosp@m..tela.nosp@m.sula.nosp@m.@gmai.nosp@m.l.co.nosp@m.m, date: August 11, 2005

Parameters

[in]	y	Argument of \(C(y)\) and \(S(y)\)
[out]	C	\(C(x)\)
[out]	S	\(S(x)\)

◆ GeneralizedFresnelCS() [1/2]

void G2lib::GeneralizedFresnelCS	(	integer	nk,
		real_type	a,
		real_type	b,
		real_type	c,
		real_type	intC[],
		real_type	intS[] )

Compute the Fresnel integrals

\[ \int_0^1 t^k \cos\left(a\frac{t^2}{2} + b t + c\right)\,\mathrm{d}t,\qquad \int_0^1 t^k \sin\left(a\frac{t^2}{2} + b t + c\right)\,\mathrm{d}t \]

Parameters

nk	number of momentae to compute
a	parameter \( a \)
b	parameter \( b \)
c	parameter \( c \)
intC	cosine integrals,
intS	sine integrals

◆ GeneralizedFresnelCS() [2/2]

void G2lib::GeneralizedFresnelCS	(	real_type	a,
		real_type	b,
		real_type	c,
		real_type &	intC,
		real_type &	intS )

Compute the Fresnel integrals

\[ \int_0^1 t^k \cos\left(a\frac{t^2}{2} + b t + c\right)\,\mathrm{d}t,\qquad \int_0^1 t^k \sin\left(a\frac{t^2}{2} + b t + c\right)\,\mathrm{d}t \]

Parameters

a	parameter \( a \)
b	parameter \( b \)
c	parameter \( c \)
intC	cosine integrals,
intS	sine integrals

◆ intersect()

void G2lib::intersect	(	BaseCurve const *	pC1,
		BaseCurve const *	pC2,
		IntersectList &	ilist )

Compute curve intersections

Parameters

[in]	pC1	first curve
[in]	pC2	second curve
[out]	ilist	list of the intersection (as parameter on the curves)

◆ intersect_ISO()

void G2lib::intersect_ISO	(	BaseCurve const *	pC1,
		real_type	offs_C1,
		BaseCurve const *	pC2,
		real_type	offs_C2,
		IntersectList &	ilist )

Compute curve intersections

Parameters

[in]	pC1	first curve
[in]	offs_C1	offset of the first curve
[in]	pC2	second curve
[in]	offs_C2	offset of the second curve
[out]	ilist	list of the intersection (as parameter on the curves)

◆ intersect_SAE()

void G2lib::intersect_SAE	(	BaseCurve const *	pC1,
		real_type	offs_C1,
		BaseCurve const *	pC2,
		real_type	offs_C2,
		IntersectList &	ilist )

inline

Compute curve intersections

Parameters

[in]	pC1	first curve
[in]	offs_C1	offset of the first curve
[in]	pC2	second curve
[in]	offs_C2	offset of the second curve
[out]	ilist	list of the intersection (as parameter on the curves)

◆ intersectCircleCircle()

integer G2lib::intersectCircleCircle	(	real_type	x1,
		real_type	y1,
		real_type	theta1,
		real_type	kappa1,
		real_type	x2,
		real_type	y2,
		real_type	theta2,
		real_type	kappa2,
		real_type	s1[],
		real_type	s2[] )

Intersect the parametric arc

\[ x = x_1+\frac{\sin(\kappa_1 s+\theta_1)-sin(\theta_1)}{\kappa_1} \]

\[ y = y_1+\frac{\cos(\theta_1)-\cos(\kappa_1 s+\theta_1)}{\kappa_1} \]

with the parametric arc

\[ x = x_2+\frac{\sin(\kappa_2 s+\theta_2)-sin(\theta_2)}{\kappa_2} \]

\[ y = y_2+\frac{\cos(\theta_2)-\cos(\kappa_2 s+\theta_2)}{\kappa_2} \]

Parameters

[in]	x1	x-origin of the first arc
[in]	y1	y-origin of the first arc
[in]	theta1	initial angle of the first arc
[in]	kappa1	curvature of the first arc
[in]	x2	x-origin of the second arc
[in]	y2	y-origin of the second arc
[in]	theta2	initial angle of the second arc
[in]	kappa2	curvature of the second arc
[out]	s1	parameter2 of intersection for the first circle arc
[out]	s2	parameter2 of intersection for the second circle arc

Returns: the number of solution 0, 1 or 2

◆ is_counter_clockwise()

integer G2lib::is_counter_clockwise	(	real_type const	P1[],
		real_type const	P2[],
		real_type const	P3[] )

Return the orientation of a triangle

Parameters

[in]	P1	first point of the triangle
[in]	P2	second point of the triangle
[in]	P3	third point of the triangle

Returns: sign of rotation

return +1 = CounterClockwise return -1 = Clockwise return 0 = flat

CounterClockwise: the path P1->P2->P3 turns Counter-Clockwise, i.e., the point P3 is located "on the left" of the line P1-P2.
Clockwise: the path turns Clockwise, i.e., the point P3 lies "on the right" of the line P1-P2.
flat: the point P3 is located on the line segment [P1 P2].

Algorithm from FileExchage geom2d adapated from Sedgewick's book.

◆ is_point_in_triangle()

integer G2lib::is_point_in_triangle	(	real_type const	point[],
		real_type const	P1[],
		real_type const	P2[],
		real_type const	P3[] )

Check if a point is inside a triangle

Parameters

[in]	point	point to check if is inside the triangle
[in]	P1	first point of the triangle
[in]	P2	second point of the triangle
[in]	P3	third point of the triangle

Returns: {0,+1,-1} return +1 = Inside return -1 = Outsize return 0 = on border

◆ minmax3()

void G2lib::minmax3	(	real_type	a,
		real_type	b,
		real_type	c,
		real_type &	vmin,
		real_type &	vmax )

inline

Return minumum and maximum of three numbers

◆ operator<<() [1/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		BBox const &	bb )

inline

Print on strem the BBox object

Parameters

stream	the output stream
bb	an instance of `BBox` object

Returns: the output stream

◆ operator<<() [2/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		Biarc const &	bi )

Print on strem the Biarc object

Parameters

stream	the output stream
bi	an instance of `Biarc` object

Returns: the output stream

◆ operator<<() [3/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		BiarcList const &	CL )

Print on strem the BiarcList object

Parameters

stream	the output stream
CL	an instance of `BiarcList` object

Returns: the output stream

◆ operator<<() [4/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		CircleArc const &	c )

Print on strem the CircleArc object

Parameters

stream	the output stream
c	an instance of `CircleArc` object

Returns: the output stream

◆ operator<<() [5/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		ClothoidCurve const &	c )

Print on strem the ClothoidCurve object

Parameters

stream	the output stream
c	an instance of `ClothoidCurve` object

Returns: the output stream

◆ operator<<() [6/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		ClothoidList const &	CL )

Print on strem the ClothoidList object

Parameters

stream	the output stream
CL	an instance of `ClothoidList` object

Returns: the output stream

◆ operator<<() [7/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		ClothoidSplineG2 const &	c )

Print on strem the ClothoidSplineG2 object

Parameters

stream	the output stream
c	an instance of `ClothoidSplineG2` object

Returns: the output stream

◆ operator<<() [8/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		Dubins const &	bi )

Print on strem the Dubins object

Parameters

stream	the output stream
bi	an instance of `Dubins` object

Returns: the output stream

◆ operator<<() [9/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		Dubins3p const &	bi )

Print on strem the Dubins3p object

Parameters

stream	the output stream
bi	an instance of `Dubins3p` object

Returns: the output stream

◆ operator<<() [10/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		LineSegment const &	c )

Print on strem the LineSegment object

Parameters

stream	the output stream
c	an instance of `LineSegment` object

Returns: the output stream

◆ operator<<() [11/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		PolyLine const &	P )

Print on strem the PolyLine object

Parameters

stream	the output stream
P	an instance of `PolyLine` object

Returns: the output stream

◆ operator<<() [12/12]

ostream_type & G2lib::operator<<	(	ostream_type &	stream,
		Triangle2D const &	t )

Print on strem the Triangle2D object

Parameters

stream	the output stream
t	an instance of `Triangle2D` object

Returns: the output stream

◆ pointInsideCircle()

bool G2lib::pointInsideCircle	(	real_type	x0,
		real_type	y0,
		real_type	c0,
		real_type	s0,
		real_type	k,
		real_type	qx,
		real_type	qy )

inline

Check if point (qx,qy) is inside the circle passing from (x0,y0) with tangent direction (c0,s0) and curvature k

Parameters

[in]	x0	starting \(x\)-coordinate of the circle arc
[in]	y0	starting \(y\)-coordinate of the circle arc
[in]	c0	\( \cos \theta \)
[in]	s0	\( \sin \theta \)
[in]	k	Curvature of the circle
[in]	qx	\( x \)-coordinate point to check
[in]	qy	\( y \)-coordinate point to check

Returns: true if point is inside

◆ projectPointOnCircle()

real_type G2lib::projectPointOnCircle	(	real_type	x0,
		real_type	y0,
		real_type	theta0,
		real_type	k,
		real_type	qx,
		real_type	qy )

Project point (qx,qy) to the circle passing from (x0,y0) with tangent direction (c0,s0) and curvature k

Parameters

[in]	x0	x-starting point of circle arc
[in]	y0	y-starting point of circle arc
[in]	theta0	initial angle
[in]	k	curvature
[in]	qx	x-point to be projected
[in]	qy	y-point to be projected

Returns: distance point circle

◆ projectPointOnCircleArc()

real_type G2lib::projectPointOnCircleArc	(	real_type	x0,
		real_type	y0,
		real_type	c0,
		real_type	s0,
		real_type	k,
		real_type	L,
		real_type	qx,
		real_type	qy )

Project point (qx,qy) to the circle arc passing from (x0,y0) with tangent direction (c0,s0) curvature k length L

Parameters

[in]	x0	\( x \)-starting point of circle arc
[in]	y0	\( y \)-starting point of circle arc
[in]	c0	\( \cos \theta_0 \)
[in]	s0	\( \sin \theta_0 \)
[in]	k	curvature
[in]	L	arc length
[in]	qx	\( x \)-point to be projected
[in]	qy	\( y \)-point to be projected

Returns: distance point circle

◆ rangeSymm()

void G2lib::rangeSymm ( real_type & ang )

Add or remove multiple of \( 2\pi \) to an angle in order to put it in the range \( [-\pi,\pi]\).

◆ solveLinearQuadratic()

integer G2lib::solveLinearQuadratic	(	real_type	A,
		real_type	B,
		real_type	C,
		real_type	a,
		real_type	b,
		real_type	c,
		real_type	x[],
		real_type	y[] )

Solve the nonlinear system

\[ A x + B y = C \]

\[ a x^2 + b y^2 = c \]

Parameters

[in]	A	first parameter of the linear equation
[in]	B	second parameter of the linear equation
[in]	C	third parameter of the linear equation
[in]	a	first parameter of the quadratic equation
[in]	b	second parameter of the quadratic equation
[in]	c	third parameter of the quadratic equation
[out]	x	\(x\)-coordinates of the solutions
[out]	y	\(y\)-coordinates of the solutions

Returns: the number of solution 0, 1 or 2

◆ solveLinearQuadratic2()

integer G2lib::solveLinearQuadratic2	(	real_type	A,
		real_type	B,
		real_type	C,
		real_type	x[],
		real_type	y[] )

Solve the nonlinear system

\[ A x + B y = C \]

\[ x^2 + y^2 = 1 \]

Parameters

[in]	A	first parameter of the linear equation
[in]	B	second parameter of the linear equation
[in]	C	third parameter of the linear equation
[out]	x	\( x \)-coordinates of the solutions
[out]	y	\( y \)-coordinates of the solutions

Returns: the number of solution 0, 1 or 2

◆ string_to_Dubins3pBuildType()

Dubins3pBuildType G2lib::string_to_Dubins3pBuildType ( string const & str )

Parameters

[in] str name of the Dubins3pBuildType type

Returns: the Dubins3pBuildType enumerator

◆ to_integer()

integer G2lib::to_integer ( DubinsType d )

Convert Dubins type solution to an integer.

Parameters

d	type of curve

Returns: a number from 0 to 5

◆ to_string() [1/2]

string G2lib::to_string ( CurveType n )

inline

Convert curve type to a string

◆ to_string() [2/2]

string G2lib::to_string ( DubinsType n )

inline

Parameters

[in] n Dubins type solution

Returns: the string with the name of the solution

◆ xy_to_guess_angle()

void G2lib::xy_to_guess_angle	(	integer	npts,
		real_type const	x[],
		real_type const	y[],
		real_type	theta[],
		real_type	theta_min[],
		real_type	theta_max[],
		real_type	omega[],
		real_type	len[] )

Given a list of \( n \) points \( (x_i,y_i) \) compute the guess angles for the \( G^2 \) curve construction.

Parameters

[in]	npts	\(n\)
[in]	x	\(x\)-coordinates of the points
[in]	y	\(y\)-coordinates of the points
[out]	theta	guess angles
[out]	theta_min	minimum angles at each nodes
[out]	theta_max	maximum angles at each nodes
[out]	omega	angles of two consecutive points, with accumulated \( 2\pi \) angle rotation
[out]	len	distance between two consecutive poijts

'

G2lib Namespace Reference

Classes

Typedefs

Functions

Detailed Description

Typedef Documentation

◆ CurveType

◆ Dubins3pBuildType

◆ DubinsType

Function Documentation

◆ build_guess_theta()

◆ collision()

◆ collision_ISO()

◆ collision_SAE()

◆ curve_promote()

◆ Dubins_build()

◆ FresnelCS() [1/2]

◆ FresnelCS() [2/2]

◆ GeneralizedFresnelCS() [1/2]

◆ GeneralizedFresnelCS() [2/2]

◆ intersect()

◆ intersect_ISO()

◆ intersect_SAE()

◆ intersectCircleCircle()

◆ is_counter_clockwise()

◆ is_point_in_triangle()

◆ minmax3()

◆ operator<<() [1/12]

◆ operator<<() [2/12]

◆ operator<<() [3/12]

◆ operator<<() [4/12]

◆ operator<<() [5/12]

◆ operator<<() [6/12]

◆ operator<<() [7/12]

◆ operator<<() [8/12]

◆ operator<<() [9/12]

◆ operator<<() [10/12]

◆ operator<<() [11/12]

◆ operator<<() [12/12]

◆ pointInsideCircle()

◆ projectPointOnCircle()

◆ projectPointOnCircleArc()

◆ rangeSymm()

◆ solveLinearQuadratic()

◆ solveLinearQuadratic2()

◆ string_to_Dubins3pBuildType()

◆ to_integer()

◆ to_string() [1/2]

◆ to_string() [2/2]

◆ xy_to_guess_angle()